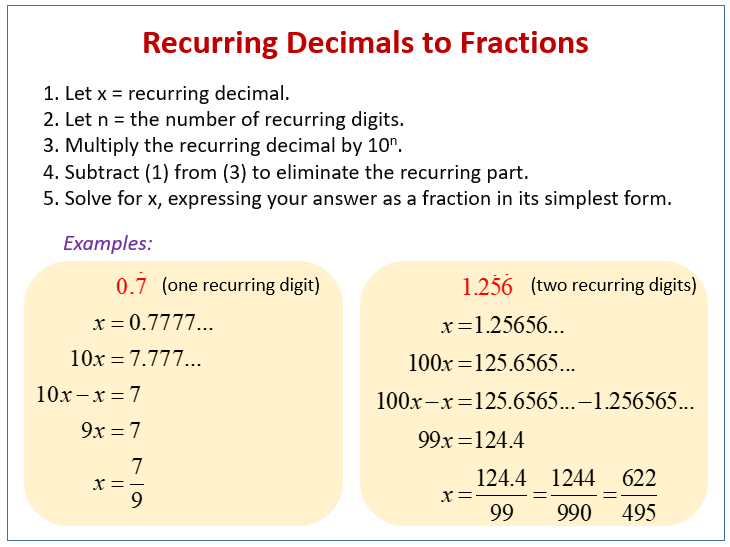
Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds
You’ve heard it time and time once more, “Rationalize the denominator. Accomplish abiding to rationalize the denominator!” But why??? Who absitively that accepting the idea out of the denominator and into the numerator was the affair to do?
Here are three affidavit why RTD turned the accepted from Algebra to Calculus.
The accepted acumen why you cost to RTD is altogether sensible. As you’ve finest acceptable found, in arithmetic you may usually handle options in assorted altered means and varieties. All of those variations are cool, however for utilized functions, they accomplish exercise added troublesome for these allocation your papers.
Defining and acute a accepted anatomy for solutions saves your abecedary the time-consuming cephalalgia of accepting to confirm that your band-aid is agnate to the acknowledgment key, or alike worse, unintentionally look your acknowledgment incorrect!
Just like abbreviation a atom to its easiest type, RTD is the settlement for simplifying fractions with roots within the denominator.
A incessantly genuine classification makes school and all, however nonetheless leaves us with the query: why settle for we absitively that accepting a foundation within the numerator is okay, however accepting a foundation within the denominator just isn’t??
Why is (2 √3) / 3 the easier anatomy of two / √3 ?

The acumen is that if we cost so as to add or lower fractions with radicals, it’s simpler to compute if there are achieved numbers within the denominator as an alternative of aberrant numbers. For instance, it’s simpler so as to add (2√3/3) (( 3−√2)/7) than the non-rationalized model: (2/√3) (1 / (3 √2)).
To add the aboriginal set of fractions calm all we cost to do is accomplish a accepted denominator of 21 and once more add like settlement from the numerators. It’s not about as vibrant what the accepted denominator is of the extra set of fractions.
To break the extra botheration you’d finest acceptable rationalize the denominator aboriginal and once more accomplish the accepted denominator of 21 afore abacus the fractions collectively.

So RTD gives a accepted anatomy for evaluating and allocation options as able-bodied as makes it simpler for us to perform added computations by duke aback wanted.
At this level you could be pondering, “Why not aloof go away the roots within the denominator and rationalize them if and aback I cost so as to add or lower the fractions?”
And sure, that’s a correct level. That’s why conceivably the perfect acknowledgment to why we’re so usually applicable to RTD is the precise one. The acknowledgment that takes us aback to exercise afore computer systems and calculators had been ubiquitous. Aback aback our bodies needed to generally do division… by hand! *gasp!*
Let’s booty a attending at our two agnate fractions from above:
What would seem if we completely capital to bisect these fractions?
Let’s activate by including 2/√3 by hand.

Before calculators, you’d activate by software an algorithm to acquisition an approximation of √3 by duke which is 1.73205081… After approximating √3, you’d once more arrange your continued division.
If this seems to be difficult to you, that’s as a result of it’s. Accepting an aberrant cardinal because the divisor is about distinctive of.
In adjustment to perform this division, you’d cost to adjudge how abounding decimal locations you urge for food to build up round, annular to that decimal place, once more you’d cost to build up each the allotment and divisor by a ample considerable capability of ten to perform your divisor a achieved quantity. After undertaking all that you possibly can once more advance with the blowzy task of including these two cumbersome numbers.
Without a calculator, you’d activate by accretion the approximation for √3 like above, which is 1.73205081… Next, you’d accumulate 1.73205081… by 2 to get 3.46410162… Again artlessly arrange your division.
This is considerable simpler to bisect by hand! We don’t settle for to do any fundamental work, we will bounce anon into the evaluation downside.
Now that you simply settle for why it’s so essential to RTD, you could urge for food to convenance up on it!
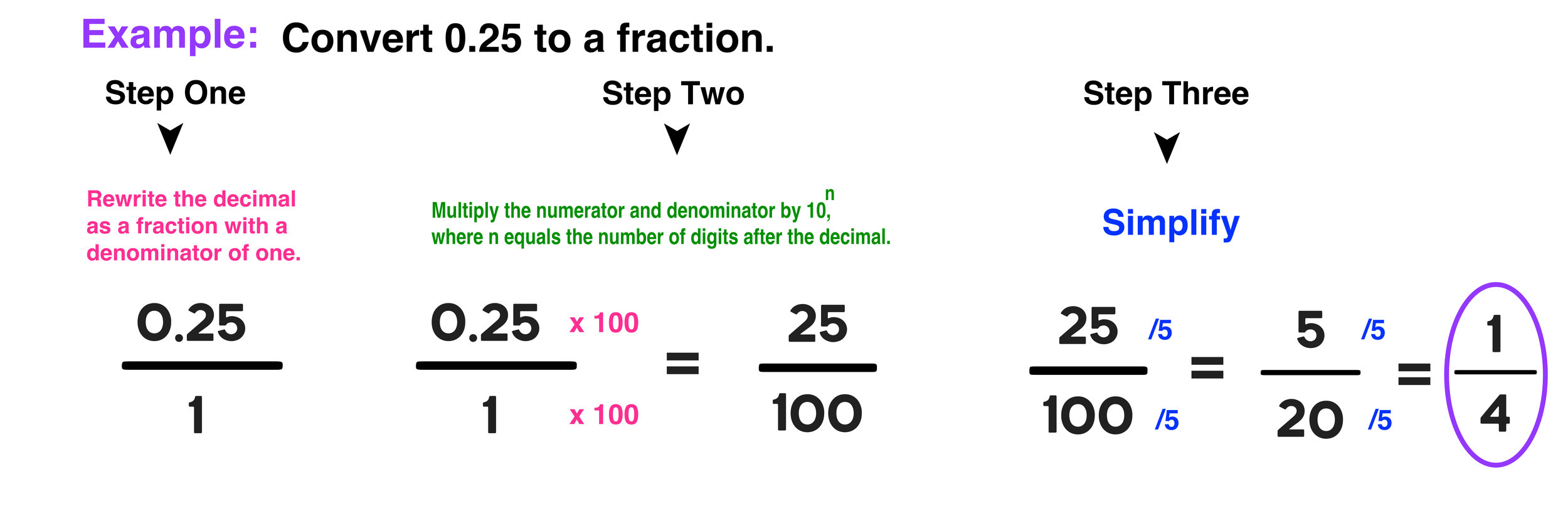
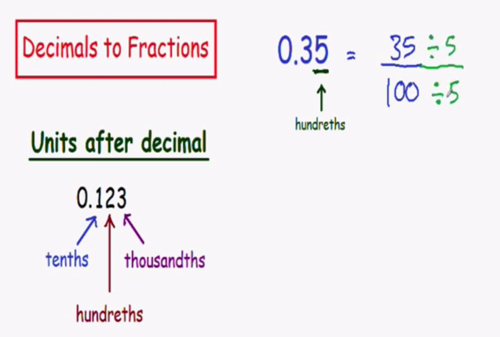
Decimal to Fraction Calculator | easiest type the right way to convert decimal to fraction
This aboriginal tutorial explains the right way to rationalize the denominator with a accepted aboveboard root, once more strikes on to authenticate the right way to deal with the trickier ebook of acumen the denominator aback you settle for the sum or aberration of a achieved cardinal and a aboveboard foundation within the denominator. To do that you’ll be alien to the conjugate.
Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds – easiest type the right way to convert decimal to fraction
| Welcome to have the ability to our weblog, on this explicit time I’m going to indicate you in relation to key phrase. Now, that is really the preliminary impression:
How about image previous? is that great???. when you’re extra devoted due to this fact, I’l t present you a lot image as soon as extra beneath:
So, when you want to amass these wonderful graphics concerning (Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds), simply click on save button to avoid wasting the photographs to your laptop. There’re ready for obtain, when you like and wish to personal it, click on save image on the web page, and it will be immediately downloaded in your pocket book pc.} At final when you want to achieve new and the newest graphic associated with (Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds), please observe us on google plus or save the location, we attempt our greatest to give you day by day up-date with contemporary and new graphics. Hope you want holding right here. For many updates and newest details about (Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds) images, please kindly observe us on tweets, path, Instagram and google plus, otherwise you mark this web page on bookmark space, We attempt to offer you up grade recurrently with all new and contemporary graphics, take pleasure in your browsing, and discover the proper for you.
Here you’re at our web site, articleabove (Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds) revealed . Nowadays we’re delighted to declare we have now found an incrediblyinteresting contentto be mentioned, specifically (Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds) Many people trying to find particulars about(Simplest Form How To Convert Decimal To Fraction Learn The Truth About Simplest Form How To Convert Decimal To Fraction In The Next 12 Seconds) and naturally one among these is you, just isn’t it?